Intermediate Sudoku Solving Techniques Part 3: Solving from Multiple Directions

In addition to focusing on one square, row or column, advanced Sudoku solving techniques often involve seeing possibilities that emerge from multiple squares, rows or columns at a time. The Sudoku grid often reveals its secrets when you’re able to see the “big picture” and find clues with a flexible mind and a clear deductive vision.

Here are some examples of how to solve Sudoku puzzles from multiple directions.

In this Sudoku grid, let’s focus on the center column (outlined in red). There are three blank spaces in this column, and by counting through the numbers which have already been placed, we know that we are missing the numbers 2, 3 and 7. If we look within each square surrounding the empty space, we can quickly get some clues – the top square contains 2 and 3 (circled in blue), the center square contains 2 and 7 (circled in blue) and the bottom square contains 7 (circled in blue).

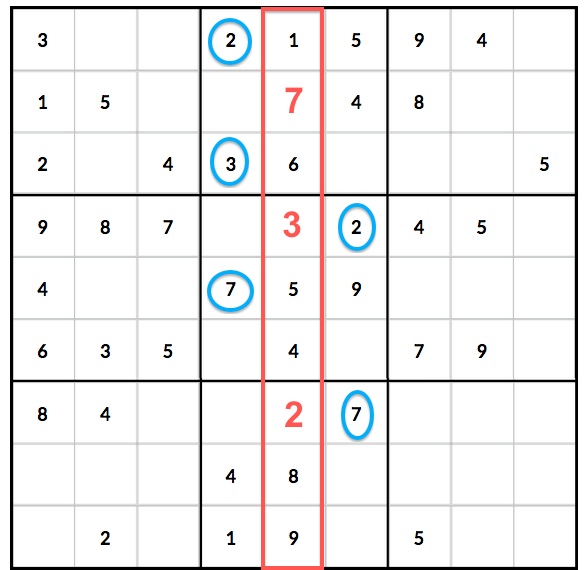
This means that we can quickly use process of elimination to fill in the blank spaces: in the top square, since we already have 2 and 3 in that square, that means the blank space must contain number 7 (marked in red).

In the center square, we already know that 2 and 7 are within that square, so the blank space must contain 3 (marked in red). And in the bottom square, there is now only one blank space remaining, and by simple process of elimination we know that it must be number 2 (marked in red).

This is an example of how to solve Sudoku spaces by looking in multiple places at once.

Another example of how “vision” can help you solve Sudoku puzzles is to look at this grid. The upper left square outlined in red contains numbers 1, 2, 3, 4 and 5 – this means that we need to fill in the spaces for 6, 7, 8 and 9. By looking to the right of the square, in the center row, we can see numbers 7, 8 and 9 – that means that the empty space in the center row cannot contain any of those numbers, and by process of elimination, must contain number 6.

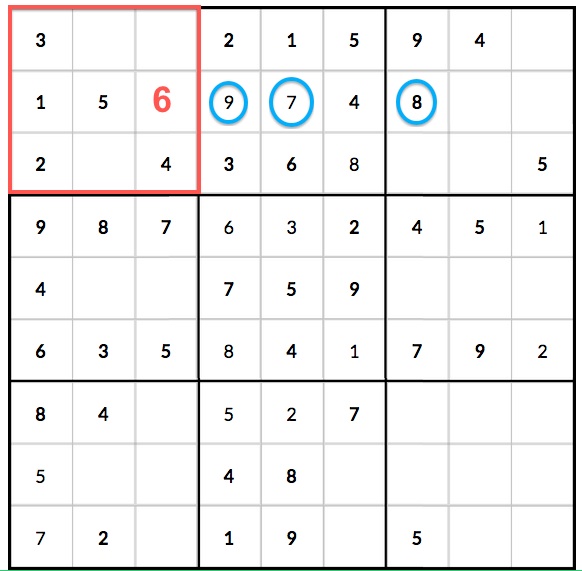
Once we have placed the number 6 in that upper left square, we can work on filling in the rest of the blank spaces in that square. This is where the “multiple directions” technique is helpful: you can see here that there are only three blank spaces left in the upper left square (outlined in red), and we know that those spaces must contain 7, 8, and 9. There is a 9 in the top row (circled in blue) and in the center column (circled in blue), which means by process of elimination, we know that neither 9 nor 8 can be placed in the space where those two rows/columns overlap – the top center space of the upper left square. This means that this space must contain number 7 (marked in green). By looking at two different rows/columns that both affected the same space of a square, we were able to eliminate those numbers from that space.

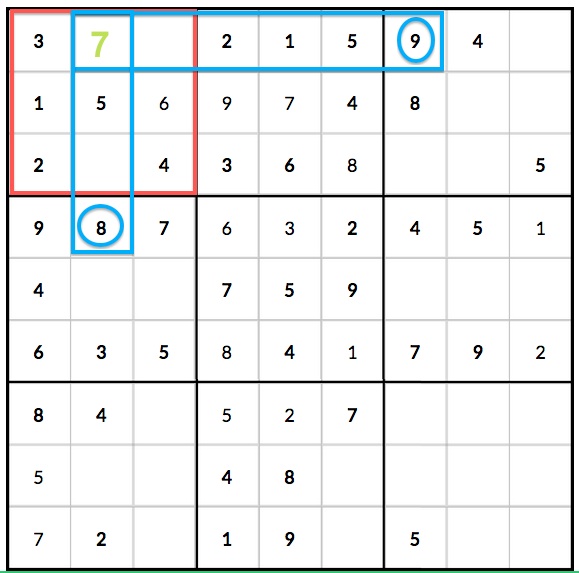
One way to get better at solving Sudoku puzzles is to expand your vision of the grid. Be able to recognize opportunities from multiple directions at once, or learn to eliminate numbers by looking at unexpected places.

What do you think about this? How have you learned to expand your vision when playing Sudoku? Leave a comment and let us know!